方龙祥，男，安徽枞阳人，教授，博士，硕士生导师。在国内外学术期刊发表论文多篇；主持和参与多项省部级项目。研究生招生方向为：统计学（学术型）、应用统计（专业硕士）。邮箱：flx529@ahnu.edu.cn

一、主要学习、工作经历和学术兼职：

1.学习经历

1997.9-2001.6 安徽师范大学数学教育专业，获理学学士学位

2004.9-2007.6 安徽师范大学概率统计专业，获理学硕士学位，师从郭大伟教授

2009.9-2014.6 复旦大学统计系，获理学博士学位，师从张新生教授

2015.1-2015.7 加拿大的Mcmaster University访学，师从Profrssor N.Balakrishnan

2.工作经历

2001.7-2011.6 安徽师范大学数学计算机科学学院，助教、讲师

2011.7-2016.6安徽师范大学数学计算机科学学院，副教授

2016.7-至今安徽师范大学数学与统计学院，教授

3.学术兼职

国内外多个学术期刊审稿人

中国运筹学会可靠性分会第十届理事会理事(2017.4-2021.12)

中国运筹学会可靠性分会第十一届理事会理事(2021.12-2025.12)

全国工业统计学教学研究会青年统计学家协会第一届理事会理事（2019.4-）

中国现场统计研究会可靠性工程分会第九届理事会理事（2021.4-）

二、主要讲授课程：

本科：概率统计、数理统计、统计建模与R软件、统计学

研究生：一般统计学、高等数理统计学、随机序、非参数统计、R软件

三、主要研究方向：

数理统计；过程统计；随机序与可靠性

四、获奖情况：

1.2019年度安徽省教学成果奖二等奖(2019jxcgj124-1):大数据背景下统计学研究生课程群的构建与成效

2.2017年度安徽省教学成果奖一等奖(2017jxcgj331-1):大数据时代背景下统计学专业实践教学改革与成效

3.2016年度安徽省教学成果奖一等奖(2016jxcgj105-7):数学建模创新人才培养模式的改革与实践

4.2019年12月获2019年度安徽师范大学教学成果奖二等奖(排名第五)

5.2018年被聘请为合肥市中级人民法院“智库专家”

6.2022.08,指导研究生参加第五届全国应用统计专业学位研究生案例大赛获得国家二等奖

7.2022.09,指导学生参加全国大学生数学建模竞赛获得国家二等奖

8.2021.6,指导学生参加安徽省大学生统计建模大赛获得本科生组一等奖

9.2022.08，指导学生参加第十二届全国大学生市场调查与分析大赛获得国家三等奖

10.2021.08，指导学生参加第十一届全国大学生市场调查与分析大赛获得安徽省分赛三等奖2项

11.2021.08，指导学生参加第十一届全国大学生市场调查与分析大赛获得安徽省分赛二等奖1项

12.2021.09，学生参加指导全国大学生数学建模竞赛获得安徽省二等奖

13.2021.11，指导学生参加安徽省大学生统计建模大赛获得本科生组二等奖1项

14.2021.11，指导学生参加安徽省大学生统计建模大赛获得本科生组三等奖2项

15.2020.12，指导学生参加安徽省大学生统计建模大赛获得本科生组市场调查分析类二等奖

16.2020.12，指导学生参加安徽省大学生统计建模大赛获得本科生组市场调查分析类优秀奖

17.2020.12，指导学生参加第十二届全国大学生数学竞赛获得非数学类三等奖2项

18.2020.09，第十届全国大学生市场调查与分析大赛安徽省分赛优秀指导教师奖

19.2020.09，指导学生参加全国大学生数学建模竞赛获获得安徽省三等奖

20.2020.06，指导学生参加第十届全国大学生市场调查与分析大赛获得国家三等奖

21.2020.06，指导学生参加第十届全国大学生市场调查与分析大赛获得安徽省分赛一等奖

五、目前主持或参与研究的主要课题：

1.安徽省哲学社会科学规划项目：基于部件相依的异构型协同系统的可靠性统计分析(AHSKF2021D30), 2021.11-2024.11，主持，在研

2.本科教学质量工程教育教学研究重点项目: 统计学专业实践教学体系的优化调整研究 ——实验课程群的构建探索(2020jyxm0682)，2021.01-2022.12

3.安徽省自然科学基金面上项目：相依单调关联系统的可靠性研究(1808085MA03),2018.6-2021.6，主持，已结题

4.安徽省科技创新战略与软科学研究专项项目：安徽省城市地下物流系统发展的研究(1706a02020010),2018.1-2019.12，主持，已结题

5.安徽省自然科学基金面上项目：无限二阶矩过程驱动的OU过程的统计推断(1408085MA07),2014.1-2017.1，主持

6.安徽省高等学校自然科学研究重点项目：非高斯Ornstein-Uhlenbeck过程的参数估计及变点检验(KJ2013A137)，2013.1-2015.12，主持

7.安徽省高等学校自然科学研究重点项目：可靠性系统里元件的分配问题研究(KJ2016A263)，2016.1-2017.12，主持

8.全国统计科学研究计划项目：基于非齐次Weibull元件的可靠性系统的研究(2012LY158)，2012.11-2014.11，主持

9.安徽省高等学校自然科学研究一般项目：不等式约束下生长曲线模型中参数的容许理论及应用(KJ2010B347)，2010.1-2011.6，主持

10.2014年校级本科课程考核改革试点项目：统计分析软件实验课程考核方式改革，主持

11.2014年校级研究生教育教学改革研究项目：统计学学科高等数理统计课程建设(2014yjg019)，主持

12.安徽师范大学博士科研启动基金项目：次序统计量的随机比较及其在可靠性理论中的应用(No. 2014bsqdjj034)，主持

13.安徽师范大学青年基金项目：不等式约束下生长曲线模型中线性估计的容许和泛容许性(2009xqn53)，2010.1-2011.6，主持

六、主要研究成果：

[1]Fang Longxiang, Zhang Xinsheng, Slepian's inequality with respect to majorization,Linear Algebra and its Applications, 434(2011) :1107-1118 (SCI)

[2]Fang Longxiang, Zhang Xinsheng, New results on stochastic comparison of order statistics from heterogeneous Weibull populations, Journal of the Korean Statistical Society, 41 (2012) :13-16 (SCI)

[3]Fang Longxiang, Slepian's inequality for Gaussian processes with respect to weak majorization,Journal of Inequalities and Applications, 5 (2013) :1-5(SCI)

[4]Fang Longxiang, Zhang Xinsheng, Stochastic comparisons of series systems with heterogeneous Weibull components,Statistics & Probability Letters, 83 (2013): 1649-1653(SCI)

[5]方龙祥,张新生,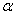-stable运动驱动的OU过程的拟似然估计[J].数学学报,57(2014): 395-408

[6]Fang Longxiang, Tang Wei, On the right spread ordering of series systems with two heterogeneous Weibull components,Journal of Inequalities and Applications, 190(2014) :1-8 (SCI)

[7]Fang Longxiang, Zhang Xinsheng,Stochastic comparisons of parallel systems with Exponentiated Weibull components,Statistics & Probability Letters, 97 (2015): 25-31(SCI)

[8]Fang Longxiang, Yang Fang, Usual multivariate stochastic order on the proportional reversed hazard rates model, Chinese Journal of Applied Probability and Statistics, 31(2015):539-546

[9]Fang Longxiang, Ling Jie, N. Balakrishnan, Stochastic comparisons of series and parallel systems with independent heterogeneous lower-truncated Weibull components, Communications in Statistics - Theory and Methods, 45(2016):540-551 (SCI)

[10]Fang Longxiang, Zhu Xiaojun, N. Balakrishnan, Stochastic comparisons of parallel and series systems with heterogeneous Birnbaum-Saunders components,Statistics & Probability Letters,112 (2016): 131-136(SCI)

[11]Fang Longxiang, N. Balakrishnan, Likelihood ratio order of parallel systems with heterogeneous Weibull components, Metrika, 79(2016): 693-703 (SCI)

[12]Fang Longxiang, N. Balakrishnan, Ordering results for the smallest and largest order statistics from independent heterogeneous exponential-Weibull random variables, Statistics, 50 ( 2016): 1195-1205 (SCI)

[13]Fang Longxiang, Barmalzan Ghobad, Ling Jie, Dispersive order of lifetimes of series systems in multiple-outlier Weibull models, Journal of Systems Science and Complexity, 29 ( 2016): 1693-1702 (SCI)

[14]Fang Longxiang, Wang Yanqin,Comparing Lifetimes of Series and Parallel Systems with Heterogeneous Fréchet Components, Symmetry , 9(2017):1-10(SCI)

[15]Fang Longxiang, N. Balakrishnan, Stochastic comparisons of series and parallel systems with generalized linear failure rate components, Applied Stochastic Models in Business and Industry, 33 (2017): 248-255 (SCI)

[16]Fang Longxiang, N. Balakrishnan, Ordering properties of the smallest order statistics from generalized Birnbaum–Saunders models with associated random shocks, Metrika, 81(2018): 19-35 (SCI)

[17]Fang Longxiang, Zhu Xiaojun, N. Balakrishnan, Stochastic ordering of minima and maxima from heterogeneous bivariate Birnbaum–Saunders random vectors, Statistics, 52 (2018):147-155 (SCI)

[18] Ding Ying, Zhang Xinsheng,Fang Longxiang, Strong modified transportation cost inequalities on k-concave probability measures with heavy tails,Statistics & Probability Letters,142 (2018): 30-38(SCI)

[19]Fang Longxiang, Zhu Xiaojun, Discussion of Birnbaum-Saunders distribution: a review of models, analysis and applications, Applied Stochastic Models in Business and Industry, 35(2019): 72-76 (SCI)

[20]Fang Longxiang, Xu Tingtao, Ordering results of the smallest and largest order statistics from independent heterogeneous exponentiated gamma random variables, Statistica Neerlandica, 73(2019):197-210 (SCI)

[21]Fang Longxiang, Cheng Meifang, Cao Daoxiang, Ding Ying, Ordering properties of largest order statistics from independent and heterogeneous Dagum populations, Communications in Statistics - Theory and Methods, 49(2020):1768-1779(SCI)

[22]Fang Longxiang, Huang Wenyu, Inequalities for Gaussian random variables under Archimedean copula dependence, Metrika,83(2020):617-625(SCI)

[23] Ling Jie,Fang Longxiang(通讯), Comparisons between largest and smallest order statistics from Pareto distributions, Journal of Mathematical Inequalities, 13(2019):1039-1055(SCI)

[24]Fang Longxiang, N. Balakrishnan, Jin Qing, Optimal grouping of heterogeneous components in series-parallel and parallel-series systems under Archimedean copula dependence,Journal of Computational and Applied Mathematics, 377(2020): 112916 (SCI)

[25]Fang Longxiang, N. Balakrishnan, Wenyu Huang, Stochastic comparisons of parallel systems with scale proportional hazards components equipped with starting devices, Journal of Industrial and Management Optimization, 18(2022): 969-984 (SCI)

[26]Fang Longxiang, Zhang Xinsheng, Jin Qing, Optimal grouping of heterogeneous components in series and parallel systems under Archimedean copula dependence, Journal of Systems Science & Complexity, 35(2022):1030-1051 (SCI)

[27]Fang Longxiang, Zhang Shuai, Lu Jinling, N. Balakrishnan, Orderings of extremes from dependent Gaussian variables with Archimedean copula under simple tree order restrictions, Statistics, 56(2022): 134-146(SCI)

[28]Fang Longxiang, Zhang Shuai, Lu Jinling, On reliability analysis in k-out-of-n systems under Archimedean copula dependence, Communications in Statistics - Theory and Methods, https://doi.org/10.1080/03610926.2022.2078840 (SCI)

[29]Fang Longxiang, N. Balakrishnan, Wenyu Huang, Zhang Shuai, Usual stochastic ordering of the sample maxima from dependent distribution-free random variables, Statistica Neerlandica, 77(2023): 99-112(SCI)

[30]方龙祥,N. Balakrishnan,黄文玉, Archimedean copula刻画的尺度比例失效率模型的极小次序统计量的随机序,数学物理学报, 2022，42：621-630

[31]何道江，盛玮芮，方龙祥，带有测量误差的Wiener退化模型的客观Bayes分析[J].应用数学学报，42(2019): 506-517.

[32]方龙祥,唐维, Fisher-Z分布次序统计量的普通多元随机序[J].数学杂志,57(2016): 395-408

[33]方龙祥，郭大伟，矩阵损失下带有不完全椭球约束的生长曲线模型中线性估计的可容许性,大学数学2008, 24: 49-53

[34]方龙祥，郭大伟，带有不等式约束的生长曲线模型中回归系数线性估计的泛容许性,数学研究2008, 41: 333-338

[35]Fang Longxiang, Guo Dawei, Admissibility of linear estimators in the growth curve Model with respect to Inequality Restriction, Northeastern Mathematical Journal, 23(2007) : 513-522